Summary
This lesson engages students in constructing the Pythagorean theorem using Cheez-It® crackers. Students will not only be able to visually see the theorem, but they will also subsequently prove why the Pythagorean theorem works for all right triangles.
Essential Question(s)
What is the Pythagorean theorem and does it work for all right triangles?
Snapshot
Engage
Students write a "preflection" about facts they know regarding triangle perimeters.
Explore
Students use Cheez-It® crackers to construct the Pythagorean theorem.
Explain
Students share their understanding and clarify misconceptions about what they explored.
Extend
Students test their newly constructed theorem on multiple right triangles for additional proof.
Evaluate
Students compare and contrast their preflection with their learning experiences.
Materials
Lesson Slides (attached)
Standard-size Cheez-It® (or similar brand) crackers (at least 50 unbroken crackers per student)
Paper
Envelopes
Card stock or construction paper
Pens or pencils
Engage
20 Minute(s)
Instruct each student to take out a blank sheet of paper. Start with slide 3 in the attached Lesson Slides.
On their paper, instruct students to engage in writing a Preflections strategy using the following prompts:
How can you tell if a triangle is a "right" triangle?
What are the legs and what is the hypotenuse in a right triangle?
If Triangle ABC has angles of A=20 degrees and C=90 degrees, how can you determine the measure of angle B?
If Triangle DEF is a right triangle, with angle F being the right angle, and side d=15 and side e=20, how do you think you could figure out the length of side f?
Feel free to use or recreate the images below to help add context to the prompts:
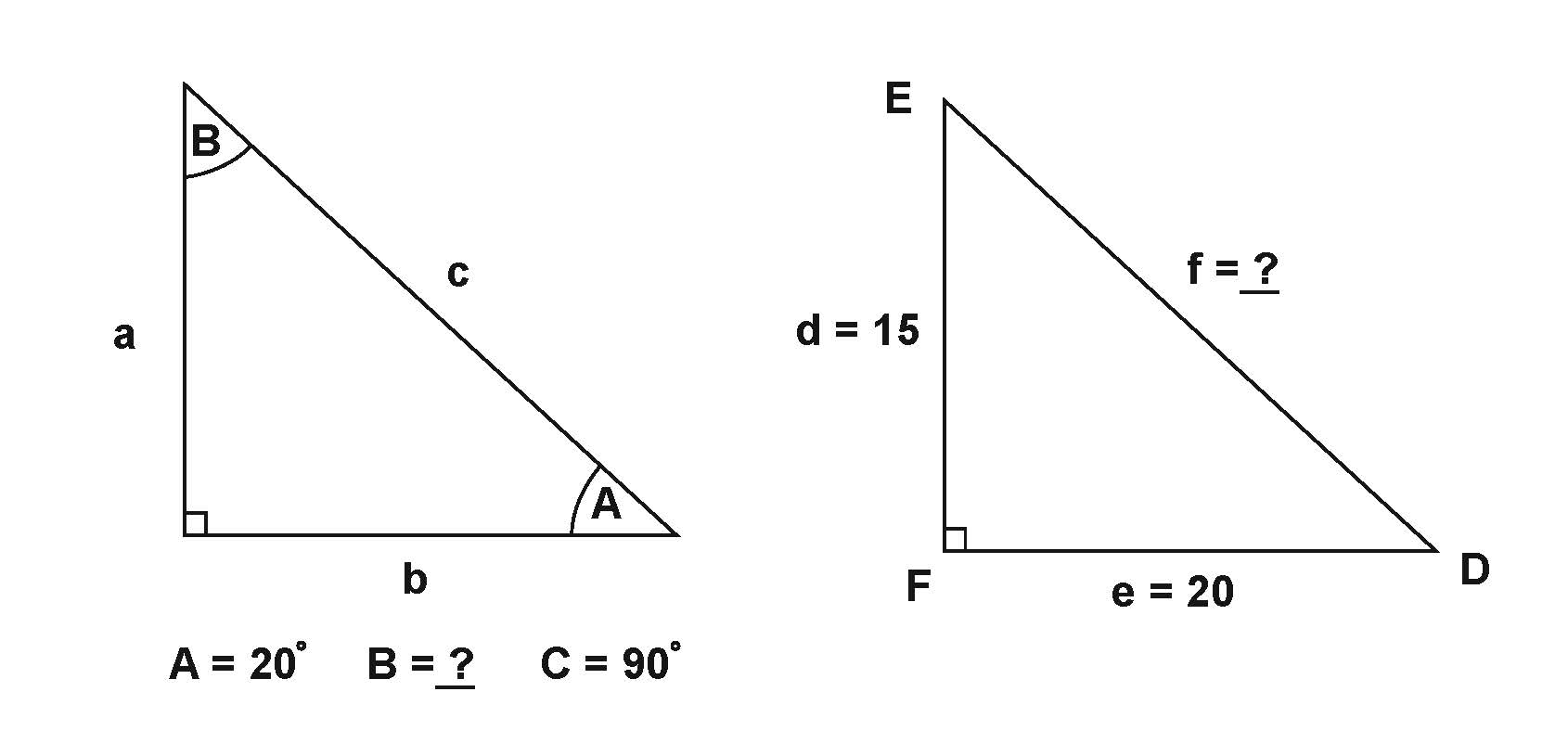
Preflections strategy prompts:
Give students approximately five minutes to write (this will feel like a long time).
While they are writing, pass out an envelope to each student. If students are struggling, remind them that these are just educated guesses and they will be able to come back to their answers later.
Ask students to ensure their name is on their paper and on their envelope.
Have students fold their paper so that it fits within the envelope, place it in the envelope, and seal it shut.
Tell students that they will revisit these at the end of the lesson, then collect all envelopes.
After students have completed their Preflections, share slide 4 and slide 5 with the essential questions and lesson objectives.
Explore
30 Minute(s)
Transition to slide 6 and guide students through the first few steps.
Guide students to:
Cut out the right triangle and place it in the center of a large piece of construction paper.
Label the two sides forming the right angle as a (shortest side) and b. Label the longest side opposite the right angle c.
Using Cheez-Its®, line the perimeter (outside edge) of the right triangle. (Note: the crackers should fit almost perfectly along the outside edges. See Fig. 1 below.)
Using the rest of the Cheez-Its®, build perfect squares outward from each side of the triangle. The perimeter will be one side of each perfect square. (See Fig. 2 below.) (Note: If needed, provide students an example: "If a side is 7 Cheez-Its® long, then build a 7 by 7 square along that edge of the triangle. Cheez-Its® will be touching but not overlapping.")
Once the perfect squares are built on all sides of the right triangle, take the Cheez-Its® from the two sides forming the right angle (sides a and b) and begin stacking these on top of the Cheez-Its® that form the square on the longest side, opposite the right angle (side c). (Note: Stack only one Cheez-It® on top of each of the existing Cheez-Its® on side c. See Fig. 3 and Fig. 4 below.)
Explain
30 Minute(s)
Trifold Instructions: Once all students have completed this activity, pass out the Pythagorean Theorem Trifold (attached). Students may now begin to complete the first page of the trifold and reflect on the two questions below the right triangle diagram.
Vocabulary and Symbols: This section of the trifold may be done before, during, or after the Cheez-It® activity. It can be used to engage prior knowledge and support students’ thinking and understanding. Once students have completed this section, share the answers provided on slides 9–10.
Transition to slide 11. Facilitate a whole class or small group discussion regarding what students notice and wonder about the Pythagorean theorem. Move to slide 12 to share the answers.
Share the video on slide 13. Let students know that the video Pythagorean Theorem [Science of NFL Football] provides an example of how this theorem could be used in real life.
Transition to slide 14 and begin the next section of the tri-fold.
Am I Right?: This section of the trifold should follow the class/small group discussion and answer students’ inquiries such as, "Does this work with any triangle or only triangles with side lengths of 3, 4, 5?" Students then begin using the Pythagorean theorem to prove and disprove if the given side lengths form a right triangle.
Reengage the whole class to discuss their findings about right triangles. Then ask students to complete the "TURN & TALK."
After a few minutes of students discussing, ask a few students to share responses to what they noticed about the relationship between the sides of the Cheez-Its® triangle, and problems 1 and 4 from the trifold.
The following "What’s My Hypotenuse?" and "What’s My Leg Length?" sections of the trifold serve as the EXTEND and might be completed during a different class.
Extend
20 Minute(s)
Move to slide 15 and begin the next section of the tri-fold.
What’s My Hypotenuse?: Instruct students to work individually or in small groups to complete problems five and six. They will also complete the WRITE, PAIR, SHARE.
Students share responses with the whole class. Through student understanding, the teacher can clear up any misunderstandings and help bridge knowledge from what students have constructed on their own exploration and experience.
Move to slide 16 and begin the next section of the tri-fold.
What's My Leg Length: Students complete the last section of the trifold. Allow students time to complete the WRITE, PAIR, SHARE and the remaining problems below. Students can share responses and solutions with other students or groups. The teacher might opt to facilitate another whole class discussion and share out.
Using the blank backside of this trifold, students can glue or tape the resource into their student notebooks.
Evaluate
10 Minute(s)
Transition to slide 17. To complete this section, distribute students' Preflection envelopes that they completed during the Engage section.
Ask students to individually open their envelopes and read through their writing.
Ask students to take out a separate sheet of paper to answer the following prompts:
What are the main differences you noticed between what you learned during the lesson compared to what you initially wrote down in your preflection?
What is the Pythagorean theorem and what is it used for?
What are two major takeaways from today's lesson that you will remember?
Resources
K20 Center. (n.d.). Preflections. Strategies. https://learn.k20center.ou.edu/strategy/191


