Summary
In this lesson, students will recall expanding polynomials and factoring quadratics. Students will learn how to factor polynomials with two, three, or four terms: difference of two squares, sum or difference of two cubes, trinomials of the quadratic form that are not quadratics, and grouping. Students will use this knowledge to factor and solve (factorable) polynomials. This lesson is not intended to be taught immediately after the Finding Factors, Part 1 lesson, but rather after students finish learning about quadratic functions.
Essential Question(s)
How are polynomial equations solved?
Snapshot
Engage
Students recall the relationship between x-intercepts of a graph and the intercept form of a quadratic.
Explore
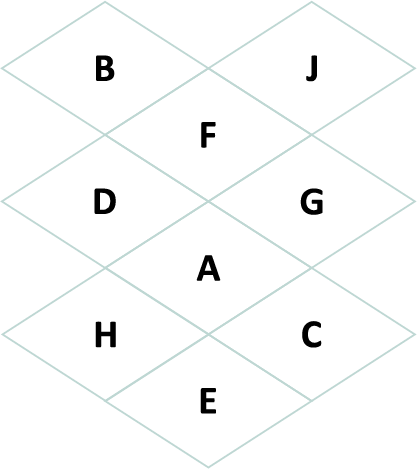
Students match expanded and factored forms of polynomial expressions to solve a diamond puzzle.
Explain 1
Students complete guided notes with the class and formalize their understanding of factoring two-term polynomials.
Extend 1
Students apply what they have learned to factor two-term polynomials.
Explain 2
Students complete guided notes with the class and solidify their understanding of factoring polynomials that are of the quadratic form or use grouping.
Extend 2
Students apply what they have learned to factor polynomial expressions and solve polynomial equations through a Choice Board.
Evaluate
Students reflect on their learning and demonstrate their understanding by creating a flowchart about the process of factoring.
Instructional Formats
The term "Multimodality" refers to the ability of a lesson to be offered in more than one modality (i.e. face-to-face, online, blended). This lesson has been designed to be offered in multiple formats, while still meeting the same standards and learning objectives. Though fundamentally the same lesson, you will notice that the different modalities may require the lesson to be approached differently. Select the modality that you are interested in to be taken to the section of the course designed for that form of instruction.
Materials
Lesson Slides (attached)
Perfect Pairings handout (attached; one per student; printed front only)
Diamond Puzzle handout (attached; one per pair; printed front only)
Guided Notes handout (attached; one per student; printed front/back)
Factor Finder handout (attached; one per student; printed front only)
Get Your Factors Straight handout (attached; one per student; printed front only)
Pencils
Paper
Scissors
Coloring utensils (optional)
Poster paper (optional)
“Finding Factors, Part 1” (optional; lesson series)
Engage
10 Minute(s)
Introduce the lesson using the attached Lesson Slides. Slide 3 displays the lesson’s essential question. Slide 4 identifies the lesson’s learning objectives. Review each of these with students to the extent you feel necessary.
Display slide 5 and give each student a copy of the attached Perfect Pairings handout. Instruct students to work individually to match each given graph with each given equation.
Move to slide 6. Using the Inverted Pyramid strategy, have students find a partner to discuss how they matched the graphs and equations. Guide students to work with their partner to then write the equation for the last graph on their handout.
After a few minutes, show slide 7 and have each pair of students find another pair of students (creating groups of four) to compare their results and reasoning.
After a few minutes, bring the class together for a whole-group discussion. Have one student from each group share their equation and reasoning with the class.
Use student responses to see if the class needs a quick review of the relationship between the intercept form of a quadratic and the x-intercepts of a parabola. If needed, guide the conversation so that students draw the logical conclusion that a polynomial could cross the x-axis more than twice and that there is a relationship between the factored form of a polynomial and its x-intercepts.
Explore
15 Minute(s)
Instruct students to find their original partner or to find a new partner. Display slide 8 and inform students that they will be practicing expanding polynomials. Pass out scissors and a copy of the attached Diamond Puzzle handout to each pair of students.
Direct students to cut out the nine diamond tiles. Have students rearrange the tiles into the pattern shown on the slide so that the sides touching are equivalent factored forms and expanded forms of the same expressions.
As students work together to solve the puzzle, use the image below as a quick way to check students’ work. This image is also available on the hidden slide 9.

Explain 1
15 Minute(s)
Display slide 10. Give each student a copy of the attached Guided Notes handout and complete the front side as a class.
After completing example 1 from the handout, which is attempting to factor x2+16, show how to factor x2–16 and then compare and contrast the two expressions. Help students understand why a sum of two squares is unfactorable.
After completing only the front side of the handout, direct students to set it aside. Students will complete the back side later in the lesson.
Extend 1
15 Minute(s)
Now it is time for students to apply what they have learned; display slide 11. Give each student a copy of the attached Factor Finder handout and direct their attention to the first part of the handout: Factoring Polynomials. Have students work with a partner of their choice or assign partners to complete this handout. Instruct students that they are to factor each expression for questions 1–2 with their partner.
As you see students finishing questions 1–2, transition through slides 12–13 so students can check their work.
Move to slide 14 and bring the class together to discuss the following questions:
What was similar about questions 1–2?
What was different about questions 1–2?
How did this impact your approach to factoring these expressions?
Display slide 15 and direct students’ attention to the second part of their handout: Solving Polynomials. As a reminder, ask the class what needs to be true about a polynomial equation before they begin factoring? If needed, use this time to review the importance of the polynomial equaling zero. Consider reminding students that quadratics are a type of polynomial, so the process of using factoring to solve will be the same as it was for quadratics.
As students progress through questions 3–6, transition through slides 16–20 so students can check their work.
Display slide 21 and bring students back together. Then, ask them the questions on the slide:
Is there a relationship between the number of solutions and the type of polynomial?
If so, what do you think it is?
Give all students a chance to consider this question. Encourage them to review their work from their handout to answer the questions. Then, ask for volunteers to share their responses.
Explain 2
20 Minute(s)
Display slide 22 and focus students’ attention on question 3 of their Factor Finder handout. Use this slide to help summarize or correct how students answered the question about the relationship between the number of solutions and the type of polynomial from the Extend 1 section of this lesson.
Move to slide 23 and use the same problem to explain the definition of “multiplicity.”
Have students get out their Guided Notes handout from earlier in the lesson and move to slide 24. Complete the back of the handout as a class.
After completing the handout, direct students to add this to their math notebook or otherwise save it according to classroom norms.
Extend 2
10 Minute(s)
Display slide 25 and introduce the Choice Board strategy. Pass out a copy of the attached Get Your Factors Straight handout to each student. Explain the directions for the activity to the class.
The handout contains nine tasks, divided into three columns (A, B, and C) and three rows (1, 2, and 3). Students are to select one task per column and one task per row, completing a total of three tasks.
In columns A and B, students are to factor the given polynomial. In column C, students are to solve the given polynomial by factoring.
Have pairs select which tasks they both want to answer.
Once students have selected their three tasks, direct students to work independently. After completing the three tasks, have students check their work with their partner.
Evaluate
20 Minute(s)
Display slide 26 and introduce students to the idea of a flowchart. The shapes in a flowchart indicate meaning, as indicated on the slide.
Give students paper (and coloring utensils if you prefer) and direct them to work with their partner to create their own flowchart that another student could use to learn how to factor polynomials. Communicate your expectations of this project with students.
Resources
K20 Center. (n.d.). Anchor Charts. Strategies. https://learn.k20center.ou.edu/strategy/58
K20 Center. (n.d.). Card Matching. Strategies. https://learn.k20center.ou.edu/strategy/1837
K20 Center. (n.d.). Choice Boards. Strategies. https://learn.k20center.ou.edu/strategy/73
K20 Center. (n.d.). Google Drawings. Tech tools. https://learn.k20center.ou.edu/tech-tool/629
K20 Center. (n.d.). Inverted Pyramid. Strategies. https://learn.k20center.ou.edu/strategy/173